
Appendix 4
Symmetrical modes and chords
Two study groups are distinguised: the symmetrical internal structures in one chord or scale and the symmetry between different chord-mode classes.
In the first group precise chords and modes are named because the symmetry will depend of the relative position of intervals.
Internal symmetries inside an octave
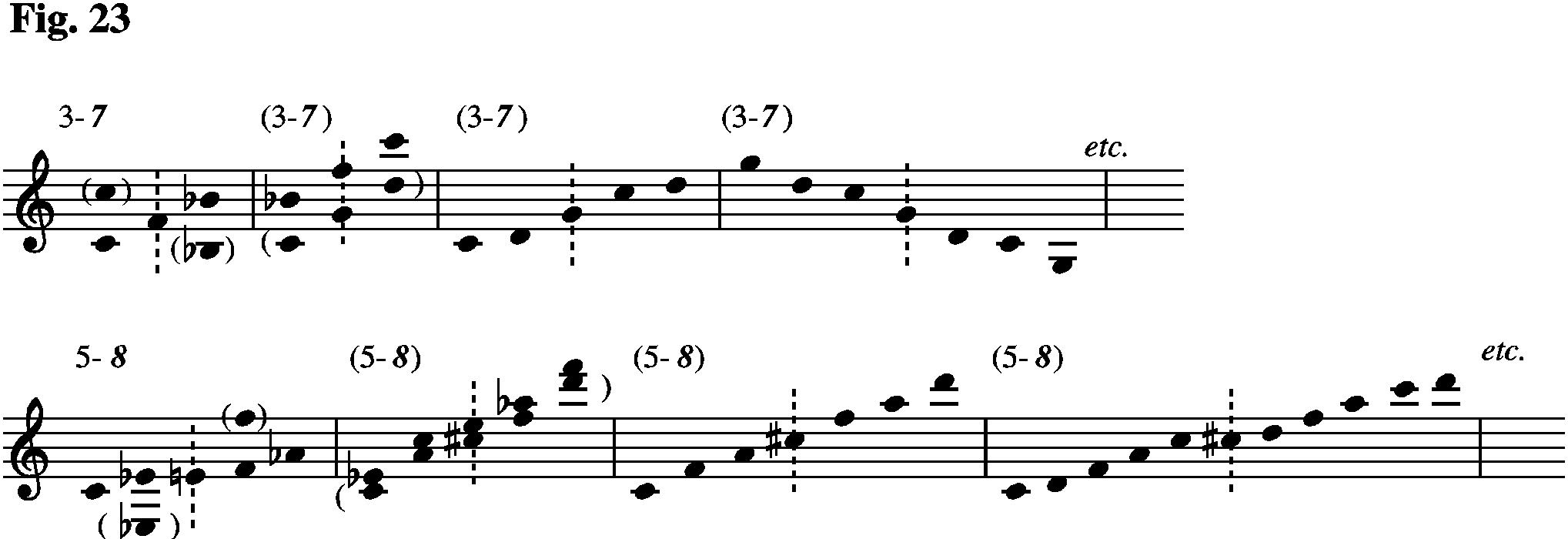
There are four different kinds of symmetry in an octave (a, b, c and d in Fig. 22): when the symmetric core goes through one or two notes and when the symmetry contemplates (or not) the closing of the octave. The numbers –in tables 6 and 7– show the quantity of pitch classes in the chord-mode class and separate by a hyphen there is the numbered classified chord-mode class in relation to the tables on pages 56-61.

A chord-mode class (except the cyclical ones) has maximum two symmetrical chords or modes –if it has one, it also has two–.

On Table 6, there are represented all the modes with symmetry from types (a) and (b) (Fig. 22) transposed to C, that is in the boundary on an octave. On table 7, there are all the modes till 7 notes with symmetry of type (c).1

As it was said at the beginning the symmetry has only been established in an octave. When the range is greater than an octave every symmetrical mode generates a great number of other symmetries (always centred in the same chord-mode class). For example:
Symmetry between chords
The subject of internal symmetries in the same chord-mode class has already been dealt with; it is now the turn to analyse the symmetry2 between different chord-mode classes. Fig. 24 shows some examples of this kind of symmetry:

A characteristic of chord-mode classes is that the symmetry of any inversion or mode of a chord-mode class is at the same time an inversion or mode of the symmetric chord-mode class. So, a chord-mode class has one and only one symmetrical chord-mode class, despite the notes layout.
Table 8 shows the relation between symmetric chord-mode classes –following the numeration on the chord-mode class tables (two first numbers)–. The third numeration is the relation with PC-Set3 (see notes on page 44). In brackets there is the real number of different symmetries –shown by an asterisk– (the symmetries that do not have an asterisk are just a repetition of a symmetry already marked –in order to facilitate their searching–). The chord-mode classes with two asterisks are doubly symmetric, they have internal symmetry and so the symmetric chord-mode is itself.4
Curiously, the number of symmetric chord-mode classes is also symmetric (1,6,12,29,38,50,38,29,12,6,1), and the same symmetry is obtained in the number of chord-mode double symmetric (1,6,5,15,10,20,10,15,5,6,1). It is just a way of showing that (CMC=chord mode class) the symmetric CMC of the complementary CMC is the complementary CMC of the symmetric CMC.
(1) From the 2048 possible modes (or chords at N5 equivalence level) 184 are internally symmetric, 63 have the symmetry of types (a) or (b) and 121 of types (c) or (d) (some cyclical modes have symmetries from the two groups); the symmetries on Table 7 are from the same chord-mode class that those on Table 6 (odd numbered groups), so the rest of symmetries until 12 notes are easily deducible.
(2) The term symmetry has been used instead of inversion to avoid misunderstanding with the different meaning this word has in the treatises on harmony in relation to chords (which is he one used).
(3) Following the numeration in the appendix 1 of The structure of Atonal Music, Forte (1973)
(4) It is the union of two symmetric chord-mode classes (from a common note).
TABLE 8
1 nota (1)
** 1 1
2 notes (6)
** 1 1
** 2 2
** 3 3
** 4 4
** 5 5
** 6 6
3 notes (12)
** 1 1 3-1
** 2 2 3-6
* 3 14 3-5
* 4 6 3-4
** 5 5 3-12
6 4
** 7 7 3-9
* 8 12 3-11
* 9 17 3-7
*10 18 3-8
**11 11 3-10
12 8
*13 19 3-2
14 3
*15 16 3-3
16 15
17 9
18 10
19 13
4 notes (29)
* 1 11 4-16
* 2 21 4-5
* 3 17 4-19
** 4 4 4-7
* 5 27 4-4
* 6 25 4-14
** 7 7 4-1
** 8 8 4-6
** 9 9 4-23
*10 23 4-22
11 1
**12 12 4-21
*13 18 4-11
*14 37 4-13
*15 31 4-27
*16 32 4-Z29
17 3
18 13
**19 19 4-24
*20 28 4-2
21 2
*22 43 4-12
23 10
**24 24 4-20
25 6
**26 26 4-8
27 5
28 20
*29 39 4-18
**30 30 4-9
31 15
32 16
**33 33 4-17
**34 34 4-3
**35 35 4-26
**36 36 4-10
37 14
*38 41 4-Z15
39 29
**40 40 4-28
41 38
**42 42 4-25
43 22
5 notes (38)
* 1 49 5-20
* 2 9 5-6
** 3 3 5-15
* 4 37 5-21
* 5 17 5-Z18
** 6 6 5-Z17
* 7 54 5-Z38
** 8 8 5-Z37
9 2
**10 10 5-22
*11 47 5-27
*12 24 5-29
*13 18 5-4
*14 45 5-2
*15 35 5-5
*16 53 5-Z36
17 5
18 13
*19 63 5-31
*20 41 5-3
**21 21 5-35
*22 48 5-23
*23 25 5-24
24 12
25 23
**26 26 5-34
*27 39 5-30
**28 28 5-33
*29 38 5-26
*30 60 5-25
*31 62 5-10
*32 66 5-28
**33 33 5-1
*34 43 5-9
35 15
*36 52 5-14
37 4
38 29
39 27
*40 46 5-13
41 20
**42 42 5-8
43 34
*44 59 5-16
45 14
46 40
47 11
48 22
49 1
**50 50 5-Z12
*51 64 5-11
52 36
53 16
54 7
*55 58 5-7
*56 61 5-32
*57 65 5-19
58 55
59 44
60 30
61 56
62 31
63 19
64 51
65 57
66 32
6 notes (50)
* 1 5 6-Z19
* 2 79 6-Z43
* 3 4 6-Z44
4 3
5 1
** 6 6 6-Z29
* 7 37 6-31
* 8 13 6-5
* 9 46 6-Z17
*10 68 6-27
*11 76 6-30
**12 12 6-Z13
13 8
*14 18 6-Z11
**15 15 6-1
*16 66 6-Z3
**17 17 6-Z6
18 14
*19 21 6-33
*20 24 6-Z24
21 19
*22 23 6-34
23 22
24 20
**25 25 6-Z23
*26 27 6-9
27 26
*28 55 6-Z12
*29 30 6-22
30 29
**31 31 6-Z4
*32 33 6-2
33 32
*34 35 6-Z10
35 34
**36 36 6-Z45
37 7
*38 48 6-15
*39 50 6-21
**40 40 6-Z49
*41 60 6-14
**42 42 6-Z28
*43 47 6-Z39
**44 44 6-Z37
**45 45 6-Z48
46 9
47 43
48 38
*49 64 6-16
50 39
**51 51 6-35
*52 65 6-Z25
*53 73 6-Z46
*54 59 6-18
55 28
**56 56 6-Z50
**57 57 6-32
*58 67 6-Z40
59 54
60 41
**61 61 6-20
**62 62 6-Z38
**63 63 6-Z26
64 49
65 52
66 16
67 58
68 10
**69 69 6-8
*70 72 6-Z47
*71 74 6-Z36
72 70
73 53
74 71
**75 75 6-Z42
76 11
*77 80 6-Z41
**78 78 6-7
79 2
80 77
7 notes (38)
** 1 1 7-35
** 2 2 7-34
* 3 4 7-32
4 3
* 5 8 7-30
** 6 6 7-33
** 7 7 7-22
8 5
* 9 24 7-14
*10 47 7-11
*11 40 7-24
*12 19 7-25
*13 60 7-23
*14 48 7-29
**15 15 7-Z12
*16 20 7-19
*17 32 7-9
*18 27 7-7
19 12
20 16
*21 42 7-27
*22 64 7-Z38
**23 23 7-Z37
24 9
*25 33 7-13
*26 41 7-Z36
27 18
*28 57 7-21
*29 30 7-6
30 29
*31 45 7-20
32 17
33 25
*34 55 7-2
*35 56 7-4
*36 43 7-5
*37 61 7-26
*38 66 7-28
**39 39 7-15
40 11
41 26
42 21
43 36
*44 59 7-Z18
45 31
*46 53 7-16
47 10
48 14
**49 49 7-8
*50 51 7-31
51 50
*52 63 7-10
53 46
**54 54 7-1
55 34
56 35
57 28
*58 65 7-3
59 44
60 13
61 37
**62 62 7-Z17
63 52
64 22
65 58
66 38
8 notes (29)
* 1 10 8-22
** 2 2 8-21
* 3 4 8-27
4 3
* 5 22 8-Z15
* 6 36 8-Z29
* 7 20 8-18
** 8 8 8-9
* 9 21 8-13
10 1
*11 34 8-16
**12 12 8-6
*13 28 8-11
*14 32 8-2
**15 15 8-8
**16 16 8-3
**17 17 8-10
**18 18 8-26
**19 19 8-17
20 7
21 9
22 5
**23 23 8-23
*24 41 8-4
*25 39 8-14
**26 26 8-24
*27 31 8-19
28 13
*29 42 8-12
**30 30 8-25
31 27
32 14
*33 35 8-5
34 11
35 33
36 6
**37 37 8-20
**38 38 8-7
39 25
**40 40 8-1
41 24
42 29
**43 43 8-28
9 notes (12)
** 1 1 9-1
* 2 6 9-8
** 3 3 9-6
* 4 12 9-11
* 5 15 9-7
6 2
** 7 7 9-10
* 8 18 6-2
* 9 11 9-5
*10 16 9-3
11 9
12 4
*13 17 9-4
**14 14 9-9
15 5
16 10
17 13
18 8
**19 19 9-12
10 notes (6)
** 1 1
** 2 2
** 3 3
** 4 4
** 5 5
** 6 6
11 notes (1)
** 1 1
12 notes (1)
** 1 1
Llorenç Balsach